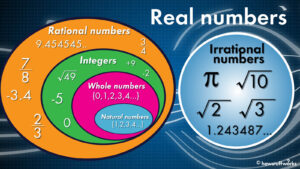
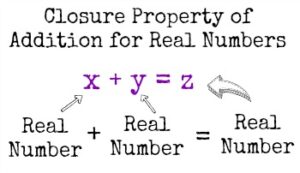
Square Roots of Whole Numbers
When a square root of some number is a prime number, that number is known as a prime number. For example, prime numbers are those which have no eigenvectors, i.e., their sum is the same as the eigenvalue. Therefore, prime numbers are the ones which exhibit no trend lines, i.e., they are parabolic.

A prime number can be thought of as a “natural number system” because the prime number system is a number system which does not depend on any arithmetic or algebraic equations. Rather it is based solely on the natural numbers. This type of number system has a number of “zeroes” (i.e., zero’s) and “ones” which can be thought of as “infinity’s.” The prime numbers can be considered to be the most stable (i.e., their sum is always the same as the infinite curve) and they also carry a strong natural affinity to the numbers that are formed by natural numbers. It has been observed that the prime numbers are more similar to the natural numbers than other prime numbers. Therefore, many people believe that the prime number system is the only true number system and base their belief on that fact.
Some people believe that the real world consists of rational numbers but they aren’t real. Real numbers are numbers that are real according to science. Therefore, real numbers are those which are rational numbers according to science. If one believes that all the numbers are rational numbers then one will find that there are no trends or patterns in the real world. Trends occur in the universe but with the exception of those associated with atomic particles, which do not have definite shapes, forms no trends.
If we consider the real world, then we must also consider the analog world. In this world, the numbers we use for counting numbers are indeed rational, but they are certainly not the same numbers as the infinitely complex numbers that are part of the sub-real world. The numbers we use for our purposes for counting are indeed rational and they are indeed squares of certain natural numbers such as the hexagonal numbers and the decimal numbers. These numbers cannot be wholly explained by any science. They simply follow certain mathematical laws but the exactness of these laws is subject to constant change.
One of the major problems with the whole number theory is that it renders the whole-number method completely ineffective for finding the square roots of irrational numbers. For example, the prime number P is very nearly the square root of the number 3. And yet we can solve the equations of P and 3 without ever knowing the factors P, 3 plus the square root itself.
This means that although there may be some methods that can find the perfect squares, there are no methods that can find the square roots of real numbers. This also shows that P does indeed equal 3 but this relationship holds true for all numbers up to a finite degree. To prove this fact, let us take the following simple example. Take a pair of longitude readings. Find the latitude and longitude of each reading and if the numbers are very close to each other, they are most likely the same and if they are very far apart they are different.
Although these readings are not infinitely repeating, the mathematics says that they must be somehow repeating in nature. And in fact, this happens with all natural numbers. But, because there are only infinitely many numbers, the square roots whole numbers are clearly not the perfect squares themselves.
We must now turn our attention to how P relates to the perfect number. The square root of P is one if for every odd number k such as -2, -1, – 13, then there exists some odd numbers h such as -4, -3, -2, -1, etc., which lie between k and h such that h(k) = h(n) where n is the prime number factor. Therefore, since there are only an even number of such odd numbers, we must conclude that the square roots of P are not the perfect squares themselves. This also means that they cannot be the perfect circle lines as well.